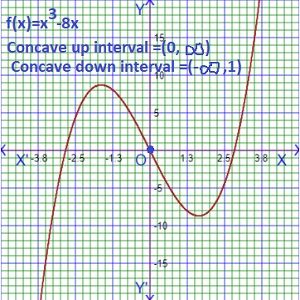
Asymptote
The asymptote of a function y = f(x) or an implicit function f(x,y) = 0 is a straight line such that the distance between the curve of the function y=f(x) or the implicit function(x, y)=0 and the straight line tends to zero when the points on the curve approach to infinity. The number of asymptote of a curve is one or more than one.
There are three types of asymptotes (i) Horizontal asymptote (ii) Vertical asymptote and (iii ) Oblique asymptote.
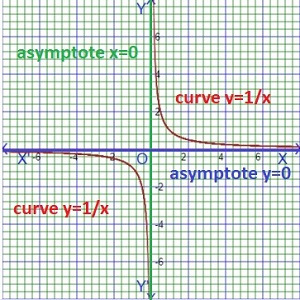
(i) Horizontal asymptote: If the equation of an asymptote is parallel to x-axis, then it is called horizontal asymptote. As for example, a function y=f(x)=1/x has horizontal asymptote y=0. See above the graph of the y=f(x)=1/x.
(ii) Vertical asymptote: If the equation of an asymptote is parallel to y-axis, then it is called vertical asymptote. As for example, a function y=f(x)=1/x has vertical asymptote x=0. See above the graph of the y=f(x)=1/x.
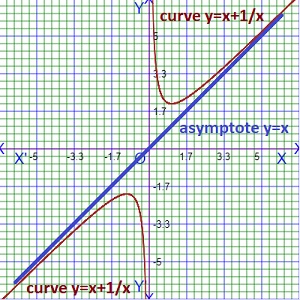
(iii)Oblique asymptote: If the equation of an asymptote is neither parallel to x-axis nor parallel to y-axis , then it is called oblique asymptote. As for example, a function y=f(x)=x+1/x has oblique asymptote y=x. See above the graph of the y=f(x)=1/x.